Introduction

In this lesson, you will learn how to calculate percentages.

This video illustrates the lesson material below. Watching the video is optional.

• Introduction to Finding Percentages (06:44 mins) | Transcript

The Clock Example

Previously, you learned about percentages with a unit that was divided into a hundred pieces. It was easy to find the percentage because it was just the number of pieces out of a hundred. The beauty of percentages is that they also show how much out of a whole thing, and that whole thing may not necessarily be divided into a hundred pieces; it may be divided into something else.

Percent means “out of 100,” but you aren’t always working with a set of 100 things. You can still calculate the percentage by taking the amount you have divided by the total amount.

For example, pretend that this is a clock.

Figure 1

Up at the top is 12 o’clock, and around the clock each hour of the day is represented: 1 o’clock, 2 o’clock, 3 o’clock, and so on. Notice the entire thing, or in other words, the clock or the time that it represents, is divided into 12 pieces. You can still figure out the percentage of time, even though time isn’t divided into segments of one hundred. In order to do this, you instead divide by the total amount, 12 in this case.

2 hours could be represented by these 2 pieces, the hours between 12 and 2.

Figure 2

If you want the percentage out of only half of the day, then it would be 12 hours. But if you want the percentage out of a whole day, that is actually 24 hours.

Twenty-Four Hours

A pie chart with 24 slices is a bit more accurate than the other version that is like a clock. You might notice that two slices are equivalent to one slice in the other chart or where it was only 12 hours. 2 out of 24 is represented by the 2 slices in this entire whole–meaning one whole thing, one whole number, or (in this case) one whole day.

Figure 3

There are 24 hours in a day, so the total amount you are comparing to is 24. To calculate the percentage of 2 hours out of a 24-hour day, divide 2 by 24:

\begin{align*} \text{Amount} \div \text{Total Amount} = 2 \div 24 = \frac{2}{24} = 0.0833 \end{align*}

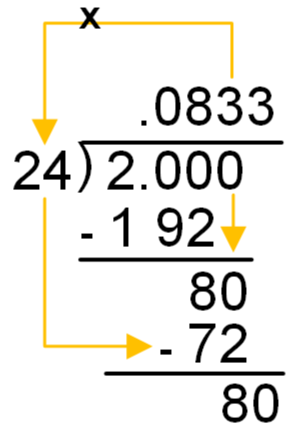
Figure 4

Then change the decimal into a percentage by multiplying by 100, or moving the decimal two places to the right, and adding a % symbol:
\begin{align*} 0.0833 \times 100 = 8.33\% \end{align*}

2 hours is 8.33% (or, if rounded, 8%) of a 24-hour day.

Things to Remember

• Use this equation to find the percent:
  \begin{align*} \text{Amount} \div \text{Total Amount} = \text{Percent} \end{align*}
• Finding a percentage is just moving the decimal over two places to the right and using a percent sign.
• Finding a percentage can be out of any number, not just one hundred. 

Practice Problems

1. What percentage is 24 minutes out of an hour? (
   Solution

   x

   Solution:
   40%
   \((24 \div 60 = 0.4 = 40\%)\)
   )
2. You are on a trip that is 292 km long. So far you have driven 88 km. What percentage of the trip have you traveled? (Round to the nearest whole percentage.) (
   Solution

   x

   Solution:
   30%
   \((88 \div 292 = 0.30137 = 30\%)\)
   Details:
   To find a percentage, calculate:
   
   \(\text{Amount} \div \text{Total Amount}\)
   
   The amount driven so far is 88 km. The total length of the trip is 292 km.
   
   \(\displaystyle \frac{\text{Amount}}{{\text{Total}} \, {\text{amount}}} = \frac{88}{292} = 0.30137...\)
   
   Change the decimal into a percentage. To do this multiply by 100, or move the decimal to the right two places, and include a % symbol:
   
   \(0.30137…=30.137\%\)
   
   Finally, round to the nearest whole percentage. Since there is a 1 in the tenths place you don’t round up.
   
   Final answer: 30%
   )
3. This month you spent $178 on food. Your total budget for the month is $1200. What percentage of the budget was spent on food? (Round to the nearest whole percentage.) (
   Solution

   x

   Solution:
   15%
   \((178 \div 1200 = 0.14833... = 15\%)\)
   )
4. You make and sell bread. The flour costs $53 a week. The cost of all your ingredients is $84 a week. What percentage of your cost is the flour? (Round to the nearest whole percentage.) (
   Solution

   x

   Solution:
   63%
   \((53 \div 84 = 0.6309… = 63\%)\)
   )
5. You take a test and get some of the extra credit points correct. You get 22 points out of 20 possible points. What is your percentage on the test? (
   Solution

   x

   Solution:
   110%
   \((22 \div 20 = 1.1 = 110\%)\)
   )
6. A water tank holds 75 L of water. There are currently 27 L in the tank. What percent of the tank is empty? (Hint: \(75-27\) is the amount missing. What percent is missing?) (
   Solution

   x

   Solution:
   64%
   \(((75-27) \div 75 = 48 \div 75 = 0.64 = 64\%)\)
   Details:
   You are looking for the percentage of water missing from the tank. Calculate this by first finding the amount of water missing: \(75-27=48\).
   
   Next, calculate:
   
   \(\displaystyle \frac{\text{Amount}}{{\text{Total}} \, {\text{amount}}} = \frac{48}{75} = 0.64\)
   
   Note: Things written in fraction form also mean division: \(\displaystyle \frac{48}{75} = 48 \div 75\).
   
   Finally, change the decimal into a percentage by multiplying by 100 and adding the % symbol. This is the same as moving the decimal two places to the right and adding the % symbol:
   
   \(0.64=64\%\)
   
   Final answer: 64%
   )

Need More Help?

1. Study other Math Lessons in the Resource Center.
2. Visit the Online Tutoring Resources in the Resource Center.
3. Contact your Instructor.
4. If you still need help, Schedule a Tutor.